
I do know how I would set these problems up given the distribution.
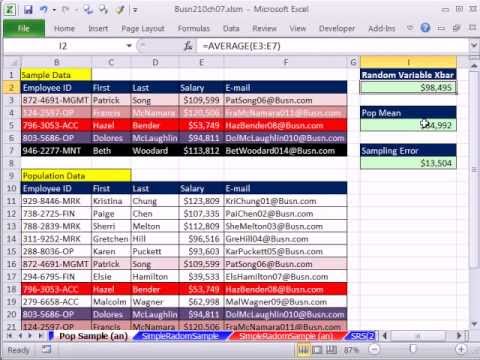
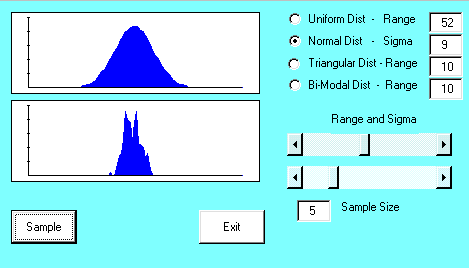
Is there anything like that for a gamma distribution? Where I can simply get the distribution of the sample mean. So far we have been mostly dealing with normal distributions and I know for a normal distribution I would divide the standard deviation by the square root of the sample size to get the distribution of the sample mean. Consider the case where two fair dice are rolled instead of one. My class is taught using only excel so I don't really have a textbook to look at or anything. Example 2: Sampling Distribution of Sample Means (x-bar). A random variable is a numerical measure of the outcome of a probability experiment whose value is determined by chance.
Sampling distribution of xbar how to#
After that, I am not sure how to incorporate the sample size into the problem. I know that I would start with the gamma distribution(1.7). The sampling distribution of X has less spread than the population distribution. Calculate the mean and standard deviation of the sampling. The sampling distribution of X is centered at the population mean. This tutorial explains how to do the following with sampling distributions in R: Generate a sampling distribution. The histogram of sample mean values is plotted with a superimposed non-normal density curve that is the theoretical sampling distribution of the sample mean and. The random variable X is the waiting time till the occurrence of the first event in a poisson process with expected waiting time beta =. A sampling distribution is a probability distribution of a certain statistic based on many random samples from a single population. Now, all we have to do is create a histogram of the values appearing in the FnofSsq column.I would really appreciate help on this homework problem.


 0 kommentar(er)
0 kommentar(er)
